1.6 $^*$Russell's Paradox

There are some logical paradoxes connected with the theory of sets. The book The Foundations of Mathematics by Evert Beth discusses 17 different paradoxes[9, pp. 481-492]. Here I discuss just one of these which was published by Bertrand Russell in 1903[43, ¶78,¶¶ 100-106].

Let $\mbox{$\cal S$}$ be the set of all sets, let $\mbox{$\cal I$}$ be the set of all infinite sets, and let $\mbox{$\cal F$}$ be the set of all finite sets. Then we have

$$\begin{array}{ccc} \mbox{$\cal F$}\in\mbox{$\cal S$}\quad & \... ...cal I$}&\quad \{\mbox{$\cal S$}\}\in\mbox{$\cal F$} \end{array}$$

Here $\mbox{$\cal F$}\in\mbox{$\cal I$}$ since there are infinitely many finite sets. $\{\mbox{$\cal S$}\}\in\mbox{$\cal F$}$ since $\{\mbox{$\cal S$}\}$ contains just one element, which is the set of all sets. Also $2\notin\mbox{$\cal S$}$ since $2$ is not a set. Next, let

$$\mbox{$\cal R$}=\{x\in\mbox{$\cal S$}\colon x\notin x\}.$$

Then for all $x\in\mbox{$\cal S$}$ we have

$$x \in {\cal R} \hspace{1ex} \Longleftrightarrow \hspace{1ex} x \notin x.\phantom{\}}$$ (1.59)

Thus

$$\begin{eqnarray*} {\cal S}\notin{\cal R}&\mbox{since}& {\cal S}\in{\cal S}, \\ ... ...f Z}\in{\cal R}&\mbox{since}& {\bf Z}\notin{\bf Z}. \phantom{\}} \end{eqnarray*}$$

We now ask whether $\mbox{$\cal R$}$ is in $\mbox{$\cal R$}$. According to (1.59),

$$\mbox{$\cal R$}\in\mbox{$\cal R$}\hspace{1ex}\Longleftrightarrow\hspace{1ex}\mbox{$\cal R$}\notin\mbox{$\cal R$},$$

i.e., $\mbox{$\cal R$}$ is in $\mbox{$\cal R$}$ if and only if it isn't!

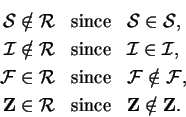
I believe that this paradox has never been satisfactorily explained. A large branch of mathematics (axiomatic set theory) has been developed to get rid of the paradox, but the axiomatic approaches seem to build a fence covered with `` keep out" signs around the paradox rather than explaining it. Observe that the discussion of Russell's paradox does not involve any complicated argument: it lies right on the surface of set theory, and it might cause one to wonder what other paradoxes are lurking in a mathematics based on set theory.

1.60   Warning. Thinking too much about this sort of thing can be dangerous to your health.

The poet and grammarian Philitas of Cos is even said to have died prematurely from exhaustion, owing to his desperate efforts to solve the paradox.[9, page 493]

Philitas was concerned about a different paradox, but Russell's paradox is probably more deadly.